Harmonic Maps Into Homogeneous Spaces
Nonfiction, Science & Nature, Mathematics, Differential Equations| Author: | Malcolm Black | ISBN: | 9781351441612 |
| Publisher: | CRC Press | Publication: | May 4, 2018 |
| Imprint: | Routledge | Language: | English |
| Author: | Malcolm Black |
| ISBN: | 9781351441612 |
| Publisher: | CRC Press |
| Publication: | May 4, 2018 |
| Imprint: | Routledge |
| Language: | English |
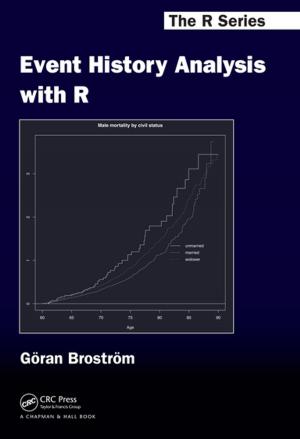
Harmonic maps and the related theory of minimal surfaces are variational problems of long standing in differential geometry. Many important advances have been made in understanding harmonic maps of Riemann surfaces into symmetric spaces. In particular, ""twistor methods"" construct some, and in certain cases all, such mappings from holomorphic data. These notes develop techniques applicable to more general homogeneous manifolds, in particular a very general twistor result is proved. When applied to flag manifolds, this wider viewpoint allows many of the previously unrelated twistor results for symmetric spaces to be brought into a unified framework. These methods also enable a classification of harmonic maps into full flag manifolds to be established, and new examples are constructed. The techniques used are mostly a blend of the theory of compact Lie groups and complex differential geometry. This book should be of interest to mathematicians with experience in differential geometry and to theoretical physicists.
Harmonic maps and the related theory of minimal surfaces are variational problems of long standing in differential geometry. Many important advances have been made in understanding harmonic maps of Riemann surfaces into symmetric spaces. In particular, ""twistor methods"" construct some, and in certain cases all, such mappings from holomorphic data. These notes develop techniques applicable to more general homogeneous manifolds, in particular a very general twistor result is proved. When applied to flag manifolds, this wider viewpoint allows many of the previously unrelated twistor results for symmetric spaces to be brought into a unified framework. These methods also enable a classification of harmonic maps into full flag manifolds to be established, and new examples are constructed. The techniques used are mostly a blend of the theory of compact Lie groups and complex differential geometry. This book should be of interest to mathematicians with experience in differential geometry and to theoretical physicists.